Spot checked the presented math on the spell calculations. Exactly correct, even on the most complicated of calculations, using multiple boost types and multiple value outputs.

Sadly, this is definitive proof that spell level is an insignificant factor in a spell’s output. Similarly, the mana mastery provided by a spell is of marginal benefit as well, but that was already called out in a previous discussion on the topic.
Thanks for the enlightenment on this matter.
In exchange, I’ll address an mechanic that is misunderstood on the spreadsheet.
On the Gear Attribute spreadsheet, this line is written,
⦿ Current Attribute Levels are determined by Item Level so a Rare, an Epic, a Legendary, and a Mythic item will all have an Attribute Level of +1 at Item Level 1.
Not all Attributes are equal at the same Item Level.
Case in point, with some examples,

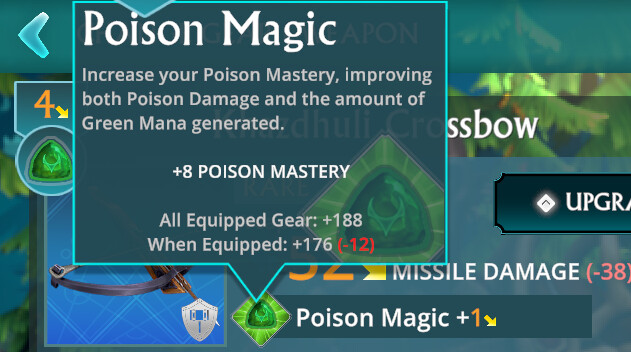
Both items are clearly at Poison Magic +1, yet the Mythic piece is offering 20 Poison Mastery, with the Rare piece offering only 8 Poison Mastery.
Why is this?
This is because the Mana Mastery system is not one-dimensional. It just seems that way because the game actually provides fast tracks for Attribute growth for Gear up to and including Legendary Rarity, if the player is of an appropriate Hero level. If the player is under-leveled, Legendary and Mythic Gear will generate an intermediate Mastery +4 result between levels 30-34, and Mythic Gear will generate an intermediate Mastery +6 result between levels 40-44.
As such, Mana Mastery is a two-variable function using both Gear Rarity, and the Player’s Hero Level as inputs.
Mathematically speaking,
- Let X = the rarity level of a piece of gear, ascending by one for each rarity tier starting at Uncommon. RarityLevel at Uncommon = 1, Rare = 2, Epic = 3, and so on.
- Let Y = the attribute level of a piece of gear.
- If the Player’s level is below 15, then AttributeLevel = 1.
- If the Player’s level is between 15 - 24, then AttributeLevel = 2.
- If the Player’s level is at least 25, then AttributeLevel is calculated as TRUNC [(Player level - 10) / 5].
The value of any Mana Mastery at any Rarity level can be modeled as,
ManaMasteryValue= 4(RarityLevel)(AttributeLevel)
Further, the Attributes Strong and Life are modeled in the same way using a similar formula.
StrongLife Value = 5(RarityLevel)(AttributeLevel)
Even further, in terms of Mana Mastery and Strong/Life Attribute Values, while their Attributes grow linearly per Attribute Level, each Rarity’s maximum Attribute Value is an exponential function, whereas each Rarity’s Attribute breakpoint is ~2.25x the maximum Attribute Value of the previous Rarity (subject to dev slight manual fudging and rounding).
For example,
Max Mana Mastery for an Rare item is 16. Max Mana Mastery for an Epic item is 16 * 2.25 = 36. Legendary gets fudged slightly at 36 * 2.25 = 81, but is shown in game as 80, as the above formula states.
As such, max Mythic Mana Mastery can be modeled as 80 * 2.25 = 180. Mythic +7 is 140 Mastery, and +8 is +160 Mastery. This lines up well for the expected 10-level Mythic range to reach this max value at +9 at level 55, right on schedule.
Food for thought, if these above models are projected further onto one more additional rarity tier beyond Mythic, a very interesting observation happens.
- Because of linear scaling of Attribute growth, max next-rarity Mana Mastery (2.25x Mythic) does not occur in 10 levels beyond Mythic. It requires 40 levels to be reached, at level 95.
Why? If one goes back and counts the Attribute levels, Rare maxes at +2. Epic maxes at +3 (+1 over Rare). Legendary maxes at +5 (+2 over Epic). Mythic will max at +9 (+4 over Legendary). Next-rarity will max at +17 (+8 over Mythic).
Notice the doubling of Attribute levels every tier over Epic? Exponential attribute/stat growth curve.
As mentioned above, there are some slight manual alterations to the models in-game made by the devs at low levels/rarities, but they hold true at higher rarities.
Hope this information helps the community.
![]()
![]()
![]()


